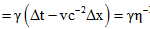J Sci Discov (2021); 5(1):jsd20041; DOI:10.24262/jsd.5.1.20041; Received August 30th, 2020, Revised October 20th, 2020, Accepted November 12nd, 2020, Published January 5th, 2021.
The Failure of Mainstream Journals and Documentaries to Eradicate the Myth of Remote Non-simultaneity:Newton Was Right and Einstein Was Wrong
Robert J. Buenker1,*
1Fachbereich C-Mathematik und Naturwissenschaften, Bergische Universität Wuppertal, Gaussstr. 20, D-42097 Wuppertal, Germany.
* Correspondence:Robert J. Buenker,Fachbereich C-Mathematik und Naturwissenschaften, Bergische Universität Wuppertal, Gaussstr. 20, D-42097 Wuppertal, Germany.E-mail: rjbuenker@gmail.com
Letter to the Editor
In July 2019 a Letter to the Editor was published in this journal [1] which spelled out the case for eliminating the claim of Remote Non-simultaneity (RNS) from the scientific literature. It was pointed out that the clocks used in making timing measurements in connection with the Lorentz transformation (LT) are inertial, that is, they are subject to no external unbalanced force. As such, their rates must be expected to maintain the same constant rate in each case indefinitely. This conclusion is closely related to Newton’s First Law of Motion (Law of Inertia) which states that every inertial object of any kind will also continue indefinitely at the same speed and in the same direction in the absence of such unbalanced forces. This characteristic of inertial clocks has been referred to as Newtonian Simultaneity and can be looked upon as a corollary to Newton’s First Law [2].
As a consequence, it has been pointed out that, by definition, the time differences Δt and Δt’ measured for the same pair of events by two different inertial clocks must always occur in the same ratio, i.e. Δt’=Δt/Q, where Q is the aforementioned ratio of clock rates. This relationship was first pointed out in 2008 [3]. It obviously makes it impossible for the clocks to disagree as to whether the events occurred simultaneously (Δt= Δt’=0) or not.
It is interesting to go back and look at the history of how the concept of non-simultaneity came about. It all started with Voigt in 1887 [4], a German scientist from Göttingen University.
He was reacting to the Michelson-Morley experiment [5] which showed that the speed of light is the same in all directions regardless of what time of the year it is. He was the first to understand that one could alter the classical (Galilean) transformation by mixing the time and space coordinates in its equations [6,7].
It was thereupon noticed by both Larmor [8] and Lorentz [9] that Voigt’s transformation did not satisfy Galileo’s Relativity Principle (RP). It has a relation between the transverse components which precludes this (Δy’=γ-1Δy, with γ=(1-v2c-2)-0.5; v is the relative speed of the two observers along their mutual x-axis and c = 299792458 ms-1 is the speed of light in free space). In order to understand why this is so, it is helpful to define the procedure of Galilean Inversion (GI). The idea is that one can form the inverse of such a relativistic equation by interchanging the primed and unprimed variables and changing the sign of the relative velocity v, i.e. what occurs when one changes the relative positions of the two observers. In the above relation, the result of GI is Δy=γ-1Δy’, which is clearly not the inverse of this equation. Larmor and Lorentz [8.9] solved this problem by eliminating the γ-1 factor in Voigt’s equation [6,7] to give simply Δy’=Δy and corresponding relation in the other perpendicular direction.
This seemed ideal since doing so preserved the Michelson-Morley equal light-speed condition, but it also resulted in the offending non-simultaneity relation of the LT:
This can be seen by considering the case when both v and Δx≠0; if the two events are simultaneous (Δt=0) in one rest frame, it is not possible to have Δt’=0 as well. When Einstein took over the LT in his landmark paper [10], he recognized that this equation forced RNS onto his theory [11]. He reconciled this frighteningly controversial prospect by inventing an example which has since been widely accepted by the physics community: two lightning strikes on a moving train [11]. He ultimately did nothing else but use the above LT equation to analyze the results of two inertial clocks, one on the train and one on the platform which it was passing, for the corresponding time differences Δt’ and Δt recorded by them. Not surprisingly, he concluded that the two clocks could not possibly agree that the lightning strikes took place simultaneously. But nowhere in the discussion is any notice taken of the fact that since the clocks are inertial, they must have perfectly constant rates and thus that their respective measured elapsed times for any pair of events must occur in exactly the same ratio as that of their constant rates.
Once the LT and its space-time mixing relation are accepted as fact, a number of other absurd predictions achieve the level of dogma in theoretical physics. Perhaps the most famous is that of symmetric time dilation. Accordingly, when two observers compare the rates of their respective in situ clocks, they must find that it is always the other’s clock that runs slower. Experimentally, this means that when they exchange light signals, each finds that the other’s is red-shifted by a factor of γ (v), where again v is their relative speed. The first attempts to observe this phenomenon involved accelerated light sources [13] and meta-stable particles such as muons [14]. In both cases, time dilation was observed, which was taken to be a verification of Einstein’s theory. A few decades later, however, experiments with rotating x-ray sources and receivers [15-17] indicated that a blue shift was observed by the accelerated receiver. In the previous experiments [13], it was the light source which was accelerated relative to the laboratory that led to the red-shift observation. Hay et al. [15] argued that the apparent inconsistency with the LT could be explained by invoking Einstein’s Equivalence Principle which he enunciated in his 1907 paper [18]. It claimed that kinetic acceleration is just another manifestation of the effects of gravity on the same object.
Sherwin had a much different interpretation, however, a short time later [19]. He concluded that the x-ray data proved conclusively that something was inconsistent with the LT explanation for the blue shift. A decade later, Hafele and Keating [20,21] carried atomic clocks onboard circumnavigating airplanes, and they found that, after excluding the effects of gravity (gravitational red shift) predicted by Einstein [18], the rate of a given clock was inversely proportional to γ(vECM), where vECM is the speed of the clock relative to the Earth’s center of mass. This fact led to the surprising result that the east-ward bound clock returned to the airport of origin with less elapsed time that that of the clock left behind there, and that the latter clock had run slower than its westward-bound counterpart. In the present context, the most interesting result is that HK found that time dilation is an asymmetric phenomenon: if one clock runs slower than the other, the latter runs faster than the first. This is the normal relationship expected for two clocks at rest on the kitchen table, and thus is in clear contradiction with the symmetric time dilation prediction of the LT.
The concept of symmetric measurement also carries over to all other physical properties such as distance and inertial mass. In the case of the former, there is an additional twist, however, which is referred to as FitzGerald-Lorentz length contraction (FLC). Not only do the observers supposedly disagree as to whose meter stick is shorter, they also find that the ratio of any two such quantities is dependent on the orientation of the object. Accordingly, the maximum contraction is observed when the object is pointed along the same direction as that of the relative velocity of the observers, where there is no difference in their measured lengths when the object is oriented in a transverse direction.
It is obvious that such relationships cannot exist in nature since both the speed of light in free space and the rates of the clocks used to measure elapsed times are perfectly independent of the orientation. The truth can be ascertained by simply considering the measurement of the light speed on a satellite. If the time it takes a light pulse to traverse a certain distance on the satellite is γ(v) times larger when the object has been accelerated on the satellite relative to its original value prior to launch, it follows that the only way the observer on the satellite could continue to measure the same value of the light speed c as found on the ground is if the meter stick on the satellite has increased in length by the same fraction. That way the corresponding measured value of the distance travelled is also γ(v) times smaller for the observer on the satellite than it was prior to launch, so that the measured ratio of distance to time is completely unaffected by the motion of the satellite (after correction for gravitational effects). In short, the slowing down of clocks due to kinetic acceleration is accompanied by length expansion in the same rest frame, not the kind of asymmetric length contraction predicted by the FLC of the LT.
Another prediction of the LT space-time mixing equation given above is time reversal. It has become of staple of science fiction movies and books. It has to do with the basic question of whether any object can travel faster than c. If a rocket travels with speed u=Δx/Δt<c, which is quite allowed in the theory, while the two observers separate with speed v>c along the same (x) axis, it is possible that uvc-2>1. If this is the case, η<0, and as a consequence, the ratio Δt/Δt’ obtained from the above LT equation is also negative.
In the real world, nobody believes in time reversal. The question remains as to how we got to this point in the first place. There are two answers, one of which is that the LT is faulty, but that has never been considered to be even a remote possibility by the physics establishment. Instead, they chose another possibility, namely that it is not allowed in nature for any object to travel at a speed greater than c. Einstein provided a solid argument in favor of this proposition in his 1905 paper [10] in connection with his E=mc2 mass/energy equivalency relation, which of course has been verified in many different experiments. He showed that the relativistic mass of a moving object is equal to γ(v) m0, where m0 is its rest mass. It therefore is eminently reasonable to conclude as a result that any system with non-zero rest mass can never attain a speed of c. This is because γ=∞ when v=c and it is impossible that the object’s relativistic mass can be unbounded.
There is a problem with the above argument, however. The speed of light in transparent media is inversely proportional to its group refractive index ng. In the neighborhood of absorption frequencies, ng<1; this would mean that the speed of light in this region exceeds c.
Sommerfeld [22] refused to accept this conclusion on the grounds that it would contradict the basic tenet that there can be no disagreement on the time order of events, i.e. Δt/Δt’<1. He claimed instead that the speed of energy transport of the waves [23] was the only quantity of experimental significance,and that its value must necessarily be less than c in all conceivable situations. This theoretical position has received wide-spread acceptance to the present day, but in 1993 new experimental evidence [24,25] emerged that appeared to demonstrate unequivocally that super-luminal (u>c) light speeds were indeed attainable in media with ng<1. However, even these results were not sufficient to dispel the general reluctance on the part of the physics community to accept as fact that single photons can indeed travel with faster-than-c speeds under the above conditions [24,26,27].
The present Letter simply asks what it will take to have the establishment in the physics community recognize that the LT is invalid and that many of its conclusions are incorrect. Experience indicates strongly that this objective has to be approached from the ground up to be successful. To this end an open letter [28] has been formulated to make the case in the most possibly compact form. It first explains what an inertial system is. Then it goes on to point out that by definition an inertial clock cannot change its rate spontaneously, as indicated in the body of the present Letter. Finally, it goes on to demonstrate that this fact precludes any possibility for the LT to be valid.
The content of the open letter was discussed on June 4, 2020 in a Zoom conference attended by representatives of 43 Indian universities. The discussion went quite smoothly and there was no objection raised to its content. The latter was provided in the form of a short power-point presentation prepared by the present author. The reader is invited to show this Letter to faculty members in physics departments with whom they are acquainted. Emphasis should be placed on departmental chairpersons and especially on colleagues who have recently given a course which contains a significant component of relativity theory.
More recently, it was noted that the journal Scientific American made a well-publicized endorsement of a presidential candidate in the upcoming U.S. election. The reason given for this unusual step (for them) was the journal’s strong belief that pollution of the atmosphere by the combustion of fossil fuels is a very significant cause of the climate change currently creating havoc in our everyday existence; it was argued their endorsement of a candidate who promised to take steps if elected which would prove very beneficial toward alleviating this problem would amount to a significant contribution to the well-being of mankind in general. Any hopes that the journal would take a similarly courageous stand with regard to Einstein’s non-simultaneity claim were dashed when a letter [29] making this point was e-mailed to them on Oct. 12, 2020. Their response was a loud roar of silence, not only to the original letter but also to a follow-up sent a week later. A link to a website [30] containing references and direct links to more than 40 related publications in peer-reviewed journals was given; the aforementioned power-point presentation was also added as an attachment.
A few months earlier a paper appeared [31] that criticized a portion of a biography of Einstein that deals with relativity theory. All attempts to contact the book’s author Prof. Walter Isaacson were in vain, similarly as with the editors of Scientific American. He is well-known for his appearances on CNN (Amanpour) and MSNBC (Morning Joe), so he is otherwise perfectly willing to air his opinions on any number of other topics. What both these attempts to obtain a useful discussion on the general subject of relativity show is that there is a great reluctance on the part of recognized authorities to engage in constructive discourse on matters of existential interest in this field.
One can add to this the experience the author had a few years back with the journal Science regarding a manuscript dealing with a closely related topic. In that case the editors were willing to at least acknowledge the correspondence but made it known that they did not believe their readership would be interested in the manuscript. They therefore declined to contact their reviewers to obtain an informed opinion. Clearly, the great majority of physicists who have ever taken a course dealing at least in part with Einstein’s version of relativity would in fact be greatly interested in a paper which proved without doubt that a central portion of it is fallacious. The editors’ position therefore can only be reasonably understood by assuming that they had come to the conclusion in private discussions among one another that what was being asserted in the manuscript could not possibly be correct, even if they weren’t able themselves to provide a cogent rebuttal thereof.
In summary, it is a travesty that for all these years students have been taught that not each pair of events which occur simultaneously for one observer will necessarily be so for another. For at least two centuries it was accepted as common knowledge that, going back to Isaac Newton, what happens in one part of the Universe occurs at exactly the same time everywhere else. Just realizing that a clock which is not subject to any unbalanced external force is incapable of changing its rate spontaneously is sufficient to show that Newton’s concept of absolute simultaneity is a Law of Physics that is not to be tampered with.
The good thing about the Truth is that no matter for how long it has been misconstrued, it is always possible to find the way back to reality simply by relying on the basic tenets of logic.
Acknowledgement of Funding Source
None
Conflict of Interest
None
References
- Buenker RJ, The myth of remote non-simultaneity: Newton was right and Einstein was wrong. J. Sci. Discov. 2019; 3(1); 1-3.
- Buenker RJ, Simultaneity and the constancy of the speed of light: Normalization of space-time vectors in the Lorentz transformation. clock puzzle and the incompatibility of proportional time dilation and remote non-simultaneity, Apeiron 2009; 16; 96-146.
- Buenker RJ, The global positioning system and the Lorentz transformation, Apeiron 2008; 15; 254-269.
- Voigt W, Ueber das Doppler’sche Princip, Goett. Nachr.1887; 41.
- Michelson AA, Morley EW, On the Relative Motion of the Earth and the Luminiferous Ether. Am . J. Sci. 1887; 34; 333. Essen L, A New Æther-Drift Experiment, Nature 1955; 175; 793.
- Buenker RJ, Voigt’s conjecture of space-time mixing: Contradiction between non-simultaneity and the proportionality of time dilation. BAOJ Physics 2017; 2:27;1-9.
- Buenker RJ, The Newton-Voigt space-time transformation. J. Sci. Discov 2019;. 3(2); 1-8.
- Larmor J, Aether and Matter, Cambridge University Press 1900; Cambridge.
- Lorentz HA, Electromagnetic phenomena in a system moving with any velocity less than that of light, Proc. K. Ak. Amsterdam 1904; 6; 809. Collected Papers, Vol. 5, 172.
- Einstein A, Zur Elektrodynamik bewegter Körper. Ann. Physik 1905; 322 (10); 891-921.
- Isaacson W, Einstein: His Life and Universe. Simon & Schuster Paperbacks 2007; New York.
- Buenker RJ, Time dilation and lightning flashes on a train. Internat. J. Applied Research 2020; 6 (9); 103-106.
- Ives WHE, Stilwell GR, An experimental study of the rate of a moving atomic clock, J. Opt. Soc. Am 1938; 28; 215-221; 1941; 31; 369-374; Mandleberg HI, Witten L, Experimental verification of the relativistic Doppler effect. J. Opt. Soc. Am. 1962; 52 (5); 529-535.
- Rossi B, Greisen K,, Stearns JC, Froman D, Koontz P. Further Measurements of the Mesotron Lifetime, Phys. Rev. 1942; 61; 675. Ayres DS, Caldwell DO, Greenberg AJ, Kenney RW, Kurz RJ, Stearns BF, Comparison of π+ and π− Lifetimes, Phys. Rev. 1967; 157, 1288.
- Hay HJ, Schiffer JP, Cranshaw TE, Egelstaff PA, Measurement of the Red Shift in an Accelerated System Using the Mössbauer Effect in Fe57, Phys. Rev. Letters 1960; 4; 165.
- Kündig W, Measurement of the Transverse Doppler Effect in an Accelerated System, Phys. Rev. 1963; 129; 2371.
- Champeney DC, Isaak GR, Khan AM, Measurement of Relativistic Time Dilatation using the Mössbauer Effect, Nature 1963; 198, 1186.
- Einstein A, Relativitätsprinzip u. die aus demselber gezogenen Folgerungen, Jahrbuch der Radioaktivität und Elektronik 1907; 4; 411-462.
- Sherwin W, Some Recent Experimental Tests of the “Clock Paradox”, Phys. Rev. 1960; 120; 17.
- Hafele JC, Keating RE, Around-the-World Atomic Clocks: Predicted Relativistic Time Gains, Science 1972; 177, 166.
- Hafele JC, Keating RE, Around-the-World Atomic Clocks: Predicted Relativistic Time Gains II, Science 1972; 177, 168.
- Sommerfeld A, Ein Einwand gegen die Relativtheorie der Elektrodynamik und seine Beseitigung, Z. Phyzik 1907; 8, 841.
- Brillouin L, Wave Propagation and Group Velocity. Academic Press 1960; New York;. 1-7.
- Steinberg AM, Kwiat PG, Chiao RY, Measurement of the single-photon tunneling time, Phys. Rev. Lett. 1993; 71, 708.
- Enders A, Nimtz G, Zero-time tunneling of evanescent mode packets, J. Phys. I France 1993; 3; 1089. Nimtz G. Schneller als das Licht?, Phys. Unserer Zeit 1997; 28; 214.
- Buenker RJ, Relativity Contradictions Unveiled: Kinematics, Gravity and Light Refraction. Apeiron. 2014; Montreal; 50.
- Buenker RJ, Faster-than-c particles and the Newton-Voigt transformation. Applied Physics ITS 2020; 3 (4); 25-35.
- Buenker RJ, Open Letter to physics departments criticizing their teaching of relativity theory. Berg. Univ. Wuppertal Library 2020; Item #62; 1.
- Buenker RJ, Letter to the Editors of Scientific American, Berg. Univ. Wuppertal Library 2020, elpub.bib.uni-wuppertal.de, Item #63; 1-4.
- Buenker RJ, Alternativelorentztransformation.blogspot.com
- Buenker RJ, Critique of the treatment of Einstein’s special theory of relativity in Isaacson’s Biography, J App. Fundamental Sci. 2020; 6 (1); 27-34 (2020
Copyright
© This work is licensed under a Creative Commons Attribution 4.0 International License. The images or other third party material in this article are included in the article’s Creative Commons license, unless indicated otherwise in the credit line; if the material is not included under the Creative Commons license, users will need to obtain permission from the license holder to reproduce the material. To view a copy of this license, visit http://creativecommons.org/licenses/by/4.0/